counter free hit unique web Download Sketch The Region Enclosed By The Given Curves. Pictures

Download Sketch The Region Enclosed By The Given Curves. Pictures

Then find the area of the region.

Download Sketch The Region Enclosed By The Given Curves. Pictures. Then find the area of the region. Find the area enclosed by the two curves.

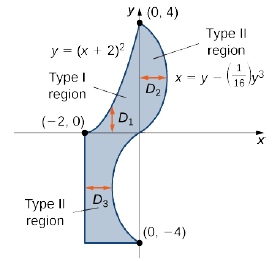
15 2 Double Integrals Over General Regions Mathematics Libretexts
15 2 Double Integrals Over General Regions Mathematics Libretexts from math.libretexts.org
*find the area of the region bounded by the curves. List the points of intersection of these curves from bottom to top in the form (x,y): From the below graph it is clear that both curves intersect each other at (2,12) and (4,24).

Full details in attached sketch.

Y = 2 x 3 , y = 1 8 x 2 , 0 ≤ x ≤ 6. Sketch the region in the first quadrant enclosed by y=6/x, y=2x, and y=12x. Decide whether to integrate with respect to x or y. Decide whether to integrate with respect to x or y to find the area a of this region.